Amplitude uniformity and asymmetry — calculations

---

This section will look at how amplitude uniformity and asymmetry are calculated. (For an introduction see Uniformity — Basic Concepts.)

Uniformity calculation (basic)

A calculated value of uniformity should have the following characteristics:

1. It should be relatively easy to determine (implement).
2. It should correlate strongly to the performance of the horn (i.e., a horn with a high uniformity should perform better than an equivalent horn with low uniformity).
3. It should allow easy comparison among various data sources (empirical amplitude measurements, FEA 3D, FEA axisymmetric, etc.).
4. It should be repeatable.

The simplest equation for uniformity is —

\begin{align} \label{eq:10301a} \widehat{U} = \frac{\overline{U}_{min}}{\overline{U}_{max}} \end{align}

where —

\( \widehat{U} \)	= uniformity
\( \overline{U}_{min} \)	= average of minimum axial amplitudes on a specified surface
\( \overline{U}_{max} \)	= average of maximum axial amplitudes on a specified surface

Notes:

1. Depending on the circumstances, uniformity can be expressed either as a decimal value or as a percent. For example, a uniformity of 0.92 is equivalent to a uniformity of 92%. Here the decimal value will generally be used.
2. Unless otherwise specified, all amplitudes used to calculate the uniformity are axial.
3. The "specified surface" will typically be the output surface. Occasionally (as will be indicated) the "specified surface" will be the input surface (e.g., when dealing with joint problems).
4. The "specified surface" may not necessarily encompass the entire surface. See Contact uniformity below.
5. \( \overline{U}_{min} \) is the average from symmetric (geometrically equivalent) locations on the specified surface where the amplitudes are a minimum; similarly for \( \overline{U}_{max} \). This is necessary because horns sometimes have asymmetric amplitude distributions, even though the horn itself is nominally symmetric with respect to the stud axis. This will become clearer below with some examples.
6. The uniformity calculated from equation \eqref{eq:10301a} will always have a value less than or equal to 1.0. (Note: the central uniformity (below) may have a value greater than 1.0.)
7. Well-designed horns should have high uniformity. Therefore, the best possible uniformity will be 1.0 (i.e., where every face amplitude is identical). The worst possible uniformity will be 0.0 when the minimum amplitude is zero. If the specified surface has a node then the uniformity is automatically zero.

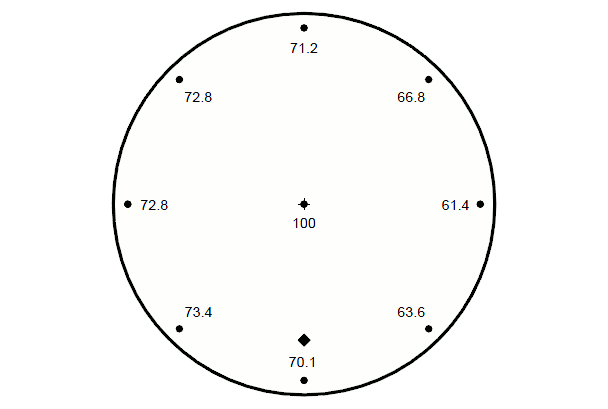
Example 1: Ø101 mm unshaped, unslotted, solid cylindrical horn

For a particular 20 kHz Ø101 mm unshaped solid cylindrical horn, the measured relative amplitudes are shown in figure 1.

Figure 1. 20 kHz Ø101 mm aluminum unshaped horn — Relative face amplitudes

According to the requirements of equation \eqref{eq:10301a}, the amplitudes must be averaged from locations on the horn that are "geometrically equivalent" — i.e., locations that would not be distinguishable from each other if the horn were rotated while an observer were blindfolded. For all unshaped cylindrical horns, the minimum face amplitude will be at the periphery. For a cylinder, any peripheral location is indistinguishable from any other peripheral location. Therefore, choosing eight equally spaced peripheral locations (a reasonable sample), the average minimum amplitude is (proceeding clockwise from the diamond reference mark):

\begin{align} \label{eq:10306a} \overline{U}_{min} &= \frac{70.1 + 73.4 + 72.8 + 72.8 + 71.2 + 66.8 + 61.4 + 63.6} {8} \\ &=69.0 \nonumber \end{align}

Thus, the average minimum amplitude (69.0) to be used in equation \eqref{eq:10301a} is greater than the least minimum amplitude (61.4).

What about the maximum amplitude? From the available data, the highest amplitude is at the center of the horn face (100). Since there is no other location on the horn that is geometrically equivalent to the center, then 100 microns is the correct value to use for the maximum amplitude.

Thus, the face amplitude uniformity is —

\begin{align} \label{eq:10307a} \widehat{U} &= \frac{69.0}{100} \\[0.3em]%eqn_interline_spacing &=0.69 \nonumber \end{align}

If the least minimum amplitude (61.4 microns) had been used in equation \eqref{eq:10301a} (rather than the averaged minimum) then the calculated (incorrect) uniformity would have been:

\begin{align} \label{eq:10308a} \widehat{U} &= \frac{61.4}{100} \\[0.3em]%eqn_interline_spacing &=0.61 \nonumber \end{align}

Number of measurements

In the above example, eight measurements were made around the periphery. Using only four measurements would have given nearly the same results. Starting at the location closest to the diamond reference mark and taking every other amplitude reading (four total), the averge minimum amplitude is 68.9 for which the uniformity is 0.689. Starting at the measurement just clockwise from the reference mark and taking every other amplitude reading (four total), the averge minimum amplitude is 69.2 for which the uniformity is 0.692. Hence, four amplitude measurements would have been sufficient for this horn.

Central uniformity

In some cases it is convenient to define a the central uniformity. In this case, the reference amplitude (in the denominator of equation \eqref{eq:10301a}) is the amplitude at the center of the chosen face, regardless if this is the largest average amplitude:

\begin{align} \label{eq:10302a} \widehat{U}_{central} = \frac{\overline{U}_{min} \textsf{ or } \overline{U}_{max}}{\overline{U}_{central}} \end{align}

Note that the stud centerline amplitude is not necessarily used for the numerator.

This definition is normally used only when all of the surface amplitudes are either larger or smaller than the centerline amplitude. If all of the amplitudes are larger than the centerline amplitude, then the uniformity will be greater than 1.0 and the surface is said to have amplitude rise. Conversely, if all of the amplitudes are smaller than the centerline amplitude, then the uniformity will be less than 1.0 and the surface is said to have amplitude droop.

This definition is especially useful for plotting graphs of uniformity versus another parameter (e.g., an altered horn dimension). See zzz for an example of this type of graph.

Contact uniformity

Some applications are welded only along in certain areas of the horn face (i.e., where the horn contacts the plastic part). Then the overall face uniformity is not important; only the uniformity over the contact area matters.

Peripheral uniformity

For a horn that contacts only around the periphery of the face, the peripheral uniformity is —

\begin{align} \label{eq:10303a} \widehat{U}_{periphery} = \frac{\overline{U}_{min~(periphery)}}{\overline{U}_{max~(periphery)}} \end{align}

zzz Example. This definition is especially useful for large cylindrical and rectangular horns.

Circular uniformity

When welding a circular part the "obvious" choice is a cylindrical horn. However, sometimes a block horn will have better performance (e.g., better uniformity over the contact area, better frequency separation, better life, etc.). When a block horn is thus used, the uniformity within the circular contact area is —

\begin{align} \label{eq:10304a} \widehat{U}_{circular} = \frac{\overline{U}_{min~(within~circle)}}{\overline{U}_{max~(within~circle)}} \end{align}

Peculiarities

Consider a peculiar situation in which the horn has rather extreme asymmetry. Figure zzz shows the amplitude distribution on the face of a 100 mm diameter spool horn. The highest face amplitude is 21.7 microns, which actually occurs at the edge of the horn face. Should we use this value as the maximum amplitude in equation \eqref{eq:10301a} to calculate the uniformity? No! Remember that you must find an average of all amplitudes at locations that are geometrically equivalent. In this case, there are seven other amplitude measurements whose locations (at the edge of the horn face) are geometrically equivalent to that of the 21.7 micron measurement. When all eight of these measurements are averaged, the result is 17.7 microns. Since this value is lower than the centerline amplitude of 19.3 microns, then 17.3 microns is the value to use in the numerator of equation \eqref{eq:10301a}, while 19.3 microns should be used in the denominator.

As the above examples show, you must be careful to use the correct values in equation ó1Â to calculate the uniformity. If asymmetry were not a problem, then you could just search the horn face for a single highest and lowest amplitude, from which the uniformity could be directly calculated. However, since most horns have some asymmetry you must take multiple amplitude measurements at geometrically equivalent locations, which are then averaged to determine the average minimum and maximum values. How many measurements are needed? This will depend on the geometry of the horn face. We will look at examples in the next sections.

Justification for averaged amplitudes

Equation \eqref{eq:10301a} uses averaged amplitudes from "geometrically equivalent locations" rather than just using the smallest and largest amplitudes. There are two reasons:

1. Using averaged amplitudes exposes certain uniformity relations that would otherwise be obscured by the horn asymmetry. In particular, it appears that the uniformity as given in equation \eqref{eq:10301a} is reasonably unaffected by the amount of horn asymmetry. (For data that supports this conclusion, see the section on "Face Uniformity for Solid, Unslotted Cylindrical Horns; Effect of Asymmetry on Uniformity".) This is not true if we simply divide the smallest measured amplitude by the largest measured amplitude.
2. When horns are machined to nominally identical dimensions, they may have somewhat different asymmetries. Using averaged amplitudes smoothes out these asymmetries so that the performance of these horns can reasonably be compared to each other or to horns of somewhat different designs.

Asymmetry

Amplitude asymmetry (generally just "asymmetry") is a measure of how much the amplitude varies at geometrically equivalent locations on the horn. Then for a horn with asymmetric amplitude, the uniformity calculation of equation \eqref{eq:10301a} only partially describes the amplitude performance. Therefore, a second parameter (asymmetry) is needed.

Asymmetry is defined as:

\begin{align} \label{eq:10305a} \textsf{Asymmetry} \widehat{A} = \frac{\textsf{Highest amplitude - Lowest amplitude}}{\textsf{Highest amplitude}} \end{align}

In this equation, both the maximum and minimum amplitude must be measured on the same surface from geometrically equivalent locations. Also, these amplitudes are the actual measured amplitudes, not averaged amplitudes.

As with uniformity, asymmetry can be expressed either as a decimal value or as a percent. For example, an asymmetry of 0.27 is equivalent to an asymmetry of 27%. Here the decimal value will generally be used.

Looking again at the above Ø100 mm horn, the amplitude is not uniform at the periphery of the horn face. With a maximum amplitude of 73.4 and a minimum amplitude of 61.4, the asymmetry is —

\begin{align} \label{eq:10309a} \widehat{A} &= \frac{73.4 - 61.4}{73.4} \\[0.3em]%eqn_interline_spacing &=0.16 \nonumber \end{align}

For well-designed horns, the best possible asymmetry will be 0.0 when all amplitudes from geometrically equivalent locations are exactly equal. The worst possible asymmetry will be 1.0 when the minimum amplitude is 0.

Limitations

Equation \eqref{eq:10305a} is not entirely adequate because it doens't consider the distance over which the asymmetry occurs. For example, consider a horn that has an asymmetry of 0.2 over the stud surface. If the diameter of the stud surface is 100 mm then the horn joint might still perform acceptably (depending on the joint amplitude). However, if the diameter of the stud surface is 40 mm then the amplitude gradient is much more severe that for the Ø100 mm horn so the horn joint should be less reliable. However, equation \eqref{eq:10305a} seems reasonable in order to avoid undue complexity. In any case, the asymmetry can simply be specified for a particular region of interest such as the joint (e.g., as \( \widehat{A}_{joint} \)).

(Note: when calculating either the uniformity or the asymmetry, use only the magnitude of the amplitudes. Do not use the sign that is associated with the phase of the amplitude. The amplitude phase is covered in the chapter on "Modeshape Analysis".)

Uniformity calculation (advanced)

Although equation \eqref{eq:10301a} is fairly easy to implement and gives consistent results, the correlation with horn performance may not be particularly good. This is because the performance depends on the amplitude distribution across the specified surface, not just the maximum and minimum amplitudes. See Appendix A.

A definition of uniformity that correlates more closely to the horn's performance is —

and where the newly defined uniformity is —

\begin{align} \label{eq:10320a} \widehat{U}' = \frac{\overline{U}} {\overline{U}_{max}} \end{align}

where —

\( \widehat{U}' \)	= uniformity (advanced)
\( \overline{U} \)	= average of all axial amplitudes on a specified surface
\( \overline{U}_{max} \)	= average of maximum axial amplitudes on a specified surface (as before)

---

Appendix A
Limitations of Equation \eqref{eq:10301a}

The following figures show hypothetical amplitude distributions for three 120 mm flat faced horn designs. Such discontinuous amplitude distributions would not be seen in actual horns but serve to show the limitations of equation \( \usetagform{} \eqref{eq:10301a} \usetagform{A} \), repeated here for convenience.

\begin{align} \label{eq:10349a} \widehat{U} = \frac{\overline{U}_{min}}{\overline{U}_{max}} \end{align}

Note that the amplitude distribution starts from the stud axis (i.e., the center of the horn's face) so the amplitude distribution goes from 0 (the stud axis) to 60 mm.

Figure A1. Horn design A — amplitude distribution

Figure A2. Horn design B — amplitude distribution

Figure A3. Horn design C — amplitude distribution

Using equation \eqref{eq:10349a} all of these horns have the same \( \overline{U}_{min} \) (50) and the same \( \overline{U}_{max} \) (100) and so all have the same uniformity (0.50). However, it seems rather obvious that horn C will perform worse than the other two horns. The performance of horn A and horn B may also be different from each other but this remains to be seen. Note: even if horn design C were transformed into design A (e.g., by machining), the calculated uniformity would not change even though the performance would have obviously have improved.

The following shows how the application performance of each horn can be evaluated numerically in terms of its ability to deliver power. First, consider that the horn face is conceptually divided into a large number n of small areas \( A_i \). Then the local power \( P_i \) that is drawn from each of these small areas is —

\begin{align} \label{eq:10351a} P_i = I_i \, A_i \end{align}

where —

\( P_i \)	= local power
\( k \)	= proportionality constant
\( A_i \)	= local area

and the local power intensity is given by —

\begin{align} \label{eq:10351a1} I_i = \frac{P_i}{A_i} \end{align}

The total power \( P \) over the entire surface of the horn is just the sum (∑) of all of the n local powers:

\begin{align} \label{eq:10352a} P = \sum{I_i \, A_i} \end{align}

It is possible that a large horn with poor uniformity may still deliver more power than a small horn having good uniformity. Therefore, to properly compare the performance of two horns having different areas, the power must be divided by the contact (face) area between the horn and the fluid. This gives the average power over the horn face — i.e., the power intensity \( \overline{I} \):

\begin{align} \label{eq:10353a} \overline{I} &= \frac{P}{A} \\[0.7em]%eqn_interline_spacing &= \left[\frac{\sum{I_i \, A_i}}{A}\right] \nonumber \end{align}

where —

\( \overline{I} \)	= average power intensity
\( P \)	= total power radiated from the face contact area
\( A \)	= total face contact area

Equation \eqref{eq:10353a} is perfectly general. Now for simplicity assume that the horn is operating in a cavitating fluid. For a cavitation load the power or intensity is directly proportional to the amplitude. (See Peshkovsky, figure 9, p. 321.) In this case equation \eqref{eq:10353a} can be written as —

\begin{align} \label{eq:10353a1} \overline{I} &= \left[\frac{\sum{(k_i \, U_i) \, A_i}}{A}\right] \end{align}

where —

\( U_i \)	= amplitude at local area \( A_i \)
\( k_i \)	= proportionality constant between amplitude \( A_i \) and intensity \( I_i \)

The proportionality constant \( k_i \) depends on factors such as the kind of fluid, the temperature and pressure, etc. For cavitation these factors can be considered to be constant across the cavitating surface. However, \( k_i \) may not be constant near the edge because of edge effects (i.e., for equal amplitudes an edge may produce less cavitation). Such possible edge effects will be ignored for this analysis of uniformity. Then \( k_i \) can be considered equal everywhere on the cavitating surface and can be moved outside the summation.

\begin{align} \label{eq:10353a2} \overline{I} &= k \, \left[\frac{\sum{U_i \, A_i}}{A}\right] \end{align}

The quantity \( \left[\frac{\sum{U_i \, A_i}}{A}\right] \) is just the average of the amplitudes \( U_i \) over the load surface (typically the face).

\begin{align} \label{eq:10354a} \overline{U} = \left[\frac{\sum{U_i \, A_i}}{A}\right]\end{align}

where —

\( \overline{U} \)

= average axial amplitude over the specified surface

Thus equation \eqref{eq:10353a1} can be written as —

\begin{align} \label{eq:10355a} \overline{I} = k \, \overline{U} \end{align}

Hence, the intensity from a cavitating load is directly proportional to the average face amplitude \( \overline{U} \).

In order to compare two horns operating at different amplitudes, \( \overline{I} \) should be divided by the horn's average maximum amplitude \( \overline{U}_{max} \). Thus, dividing both sides of equation \eqref{eq:10355a} by \( \overline{U}_{max} \) gives:

\begin{align} \label{eq:10356a} \overline{I}' &= \frac{\overline{I}}{\overline{U}_{max}} \\[0.7em]%eqn_interline_spacing &= k \, \frac{\overline{U}} {\overline{U}_{max}} \nonumber \\[0.7em]%eqn_interline_spacing &= k \, \widehat{U}' \nonumber \end{align}

where —

\( \overline{I}' \)	= average intensity per unit amplitude
\( \overline{U}_{max} \)	= average of maximum axial amplitudes over the specified surface

and where the newly defined uniformity is —

\begin{align} \label{eq:10357a} \boxed{ \widehat{U}' = \frac{\overline{U}} {\overline{U}_{max}} } \end{align}

This uniformity is designated as \( \widehat{U}' \) to distinguish it from the original \( \widehat{U} \) of equation \eqref{eq:10349a}. This definition is very similar to equation \eqref{eq:10349a} except that the numerator \( \overline{U} \) is the average amplitude over the entire horn face rather than the average of the face amplitude minimums \( {U}_{min} \). Now, however, this definition satisfies the requirement that the horn's performance should correlate to the uniformity — i.e., for two horns with the same normalized maximum face amplitudes, the one with the greatest uniformity \( \widehat{U}' \) will have the highest average power intensity \( \overline{I}' \).

Comparison to equation \eqref{eq:10349a}

For the above hypothetical horns of figures A1 through A3, the assumed horn shape could either be rectangular or cylindrical. The calculated uniformities from equations \eqref{eq:10349a} and \eqref{eq:10357a} are shown in table A1 and are discussed below.

Table A1. Comparison of uniformity calculations

Horn Horn shape \( \widehat{U} \) - equation \eqref{eq:10349a} \( \widehat{U}' \) - equation \eqref{eq:10357a} A Rectangular 0.5 0.958 B Rectangular 0.5 0.958 C Rectangular 0.5 0.750 A Cylindrical 0.5 0.921 B Cylindrical 0.5 0.995 C Cylindrical 0.5 0.667

When equation \eqref{eq:10349a} is used to evaluate the uniformity, all of the horns have the same uniformity, regardless of the amplitude distribution or the horn shape. Therefore, equation \eqref{eq:10349a} gives no indication of which horn will perform best. In contrast, the uniformity from equation \eqref{eq:10357a} helps to distinguish the horns according to their performance.

Rectangular horns

For a rectangular horn the local area \( A_i \) in equation \eqref{eq:10354a} is just \( t \, Δx \) where \( t \) is the face thickness and \( Δx \) is a small increment along the horn's width. (For simplicity here, the horn's amplitude is assumed to be constant across the face thickness.) Then horns A and B have equal uniformities (0.958) and will deliver equal cavitation power, whereas horn C is less uniform (0.750) and will deliver lower cavitation power.

Cylindrical horns

For a cylindrical horn the local circular area \( A_i \) in equation \eqref{eq:10354a} is \( 2π r Δr \) where \( r \) is the radius where \( A_i \) is being evaluated and \( Δr \) is a small increment centered at \( r \). Even though all of the cylindrical horns have the same physical face area, the delivered power depends not only on the local amplitude but also on the local circular area where the power is delivered. Horn B has 100 relative amplitude at its periphery where the circular area of the horn is greatest. In contrast, horn A has lower relative amplitude in this critical region. (Horn A does have better relative amplitude near the stud axis but this region is less effective because the circular area there is less.)

Thus, horn B has the highest uniformity (0.995) followed by horn A (0.921). Horn C again finishes last but its uniformity as calculated from equation \eqref{eq:10357a} (0.667) is still better than that from equation \eqref{eq:10349a} (0.5).

To get a more intuitive feel for the cylindrical horns, consider cylindrical buckets that are each filled with sand to a maximum height h. The sand at the top of each bucket is distributed in the same manner as the amplitude distribution. The bucket with the most sand (the heaviest bucket) will come closest to the "ideal" bucket where the sand is completely level across the top. Bucket B will have the most sand, followed by bucket A and then bucket C. In fact, bucket B will have 99.5% as much sand as a bucket whose sand is completely level.

Special case - all \( A_i \) are equal

There is a special case for equation \eqref{eq:10354a} where all of the small local areas \( A_i \) are equal; call this small area \( A_0 \). Since \( A_0 \) is fixed it does not need to be associated with a particular \( U_i \) and so can be moved outside the summation. Thus, equation \eqref{eq:10354a} becomes:

\begin{align} \label{eq:10358a} \overline{U} &= \frac{A_0\sum{U_i}}{A} \\[0.7em]%eqn_interline_spacing &= \frac{\sum{U_i}}{(A/A_0)} \nonumber \end{align}

\( A/A_0 \) is just the total number \( n \) of locations where the amplitude is to be evaluated. Thus, equation \eqref{eq:10358a} becomes:

\begin{align} \label{eq:10359a} \overline{U} &= \left( \sum{U_i} \right)/n \end{align}

The advantage of equation \eqref{eq:10359a} over equation \eqref{eq:10354a} is that the individual local areas \( A_i \) don't have to be considered. In fact, equation \eqref{eq:10359a} is completely independent of the horn contact area. Thus, for scanning laser vibrometer where the measurement points are equally spaced or FEA (for the case where the mesh is relatively uniform), the uniformity can be calculated reasonably easily by using equation \eqref{eq:10359a} in equation \eqref{eq:10357a}:

\begin{align} \label{eq:10360a} \widehat{U}' &= \frac{\left( \sum{U_i} \right)/n} {\overline{U}_{max}} \end{align}

Implementation problems

Although equations \eqref{eq:10357a} and \eqref{eq:10360a} are conceptionally appealing, they may be difficult to implement in practice.

Experimental

For experimental measurements, one of the main problems is the large number of amplitude \( U_i \) data that must be obtained in order to determine the average face amplitude \( \overline{U} \), especially if the horn has any amplitude asymmetry. For example, for a 20 kHz horn assume that the amplitude must be determined at grid spacings of 10 mm. (This may actually be too large but can serve for discussion.) The following table shows the required number of amplitude measurements for various horns.

Table A2. Required number of amplitude measurements depending on horn dimensions

Horn dimensions Horn type Required number of amplitude measurements 200 mm x 15 mm Bar 20 200 mm x 60 mm Bar 120 200 mm x 200 mm Block 400 Ø200 mm Cylindrical 300

The first horn in the table is a bar horn. Since the 15 mm face thickness is relatively small, the amplitude should not vary much across the thickness. Then amplitude measurements would only be needed at 10 mm increments across the horn's width. This could reasonably be done by manually positioning an amplitude probe.

The remaining horns have significant lateral dimensions so the amplitudes would need to be determined across the entire face. This would not be reasonable for a manually positioned probe. Then some kind of whole-field measurement method would be needed.

(The above table assumes that amplitude measurements are needed over the entire horn face, possibly due to amplitude asymmetry. If asymmetry is assumed to be negligible then the amplitudes only need to be measured on a subsection of the horn's face. For example, only a quarter section might suffice.)

Direct amplitude measurement

Equation \eqref{eq:10357a} was derived based on the ability to correlate horn uniformity with cavitation loading in water. Hence, the relative uniformity of two horn designs might be compared by the following procedure.

1. Measure the power of the ultrasonic stack in air.
2. Immerse the horn face in degassed room-temperature water of a suitable container.
3. Increase the amplitude (as needed) until full cavitation occurs over the horn face.
4. Measure the power.
5. In air with the same setting on the power supply, measure the horn's highest average amplitude.
6. Calculate the intensity from equation \eqref{eq:10357a} or \eqref{eq:10360a}.

This procedure has several difficulties.

1. This procedure does not give an absolute measure of uniformity. Instead, it gives an intensity value that correlates with the uniformity. Hence, it is only useful for comparing the relative performance of different horns.
2. The power in water derives not only from the horn's axial amplitude but also from any transverse amplitude at the periphery where the horn is immersed. Thus, the power that is dependent only on the axial uniformity of the face amplitude can't be independently determined (unless the transverse amplitude is small in which case the cavitation power due to transverse amplitude can be neglected).
3. Equation \eqref{eq:10353a2} and subsequent equations were derived under the assumption that edge effects could be neglected. Such effects are likely but their relative effect is unknown (except that they may be more pronounced in smaller horns where the ratio of edge to face surface area is larger).
4. Since the entire horn face must be immersed, this procedure is not suitable for measuring the uniformity over a limited face area.
5. The power supply must have sufficient power to induce cavitation which may be a problem when evaluating larger horns.
6. Care must be taken that other horn entities such as slots, flutes, etc. are not submerged since these will produce additional cavitation power that can't be accounted.
7. The reproducibility of this method is questionable, especially when measurements are made at different facilities with different equipment.

Analytical (computer simulation)

For computer simulation by FEA, the amplitude values can easily be taken from the FEA mesh nodes. However, difficulties remain.

1. Equation \eqref{eq:10360a} only applies when the locations of amplitude measurement are equally spaced from one another so that all of the local \( A_i \) are the same. For FEA there are many situations where this is not true. For example, when slots are reasonably close to the face, the face mesh near the slot ends will be finer than the surrounding mesh. Then equation \eqref{eq:10360a} can't be used because the finer local mesh would have undue influence; instead, equation \eqref{eq:10357a} must be used. However, equation \eqref{eq:10357a} involves the average amplitude \( \overline{U} \) (equation \eqref{eq:10354a}) which can only be evaluated if the associated local areas \( A_i \) can be determined for each local amplitude \( U_i \). However, the \( A_i \) are typically not available directly from the FEA because the nodes themselves don't have areas; only the element faces have areas. However, the required \( A_i \) might be obtained by massaging the raw FEA data (e.g., by computing \( A_i \) from the element faces that are adjacent to node i).
2. When FEA analyzes only a subsection of the horn (for example, a quarter section of a cylindrical horn), equation \eqref{eq:10357a} would give full weight to the amplitudes at nodes at the subsection boundary. In fact, these amplitudes should only be given half-weight since these nodes in the full model are actually shared between the adjoining sections. Thus, the uniformity calculated using equation \eqref{eq:10357a} for a subsection will not agree with the uniformity if equation \eqref{eq:10357a} had been applied to the entire horn face. However, the descrepency will be small as long as the number of boundary nodes is small compared to the total number of face nodes. (A similar argument can be made for nodes at the periphery of the horn face since the associated area of such nodes is only half that of interior nodes.)
3. For an axisymmetric FEA model, the entire face is not available. Instead, the amplitudes are only available along a single radial line across the face. In order to evaluate the uniformity, the circumferential area associated with each node must be calculated as:

   \begin{align} \label{eq:10361a} A_i =2\pi \, r_i \, w_i \end{align}

   where —

   \( A_i \)	= circumferential area of the element at node i
   \( r_i \)	= radial distance of node i from the face centerline
   \( w_i \)	= radial width of the element at node i

   Each \( w_i \) can be calculated as an average, based on the radii of the adjacent nodes (\( r_{i+1} \) and \( r_{i-1} \)):

   \begin{align} \label{eq:10362a} w_i &= \frac{r_{i+1} + r_i} {2} - \frac{r_{i} + r_{i-1}} {2} \\[0.7em]%eqn_interline_spacing &= \frac{r_{i+1} - r_{i-1}} {2} \nonumber \end{align}

   where —

   \( r_{i+1} \), \( r_{i-1} \)

   = radii of nodes adjacent to node i

   Special consideration must be given to the centerline node and the node at the periphery of the face.

   Equation \eqref{eq:10361a} may best be calculated by exporting the axisymmetric amplitudes and associated radii to external software (e.g., a spreadsheet) and performing the calculations there.

Thus, although equation \eqref{eq:10357a} is conceptionally better than equation \eqref{eq:10349a}, it may not be practical due to the above problems.